The Beer-Lambert Law

Summary:

The text discusses the Beer-Lambert Law, which explains the relationship between the absorption of light and the properties of the material through which it passes. It explores the terms absorbance and molar absorptivity in the context of UV-visible absorption spectrometry.

According to the Beer-Lambert Law, the absorbance of a solution is directly proportional to its concentration and the length of the light path through the solution. The law is expressed as A = εcl, where A is the absorbance, ε is the molar absorptivity, c is the concentration, and l is the length of the light path.

The molar absorptivity measures the likelihood of electronic transitions and is expressed in units of L mol⁻¹ cm⁻¹. It allows for comparisons between different compounds under standardized conditions. The absorbance can vary depending on concentration and the length of the light path.

The text highlights the importance of concentration in determining the extent of light absorption and emphasizes the need to consider both concentration and path length when comparing absorbance values. It also discusses the concept of molar absorptivity and how it accounts for variations in concentration and path length.

Overall, the topic discussed in the text is related to analytical chemistry and spectroscopy, specifically the Beer-Lambert Law and its application in determining the absorbance of solutions using UV-visible absorption spectrometry.

Excerpt:

The Beer-Lambert Law

The Beer-Lambert law relates the constriction of light to the properties of the material through which the light is voyaging. This page investigates the Beer-Lambert Law and makes sense of the utilization of the terms absorbance and molar absorptivity connecting with UV-noticeable assimilation spectrometry

The Absorbance of a Solution
For every wavelength of light going through the spectrometer, the power of the light going through the reference cell is estimated. This is typically alluded to as Io – that is, I for Intensity.

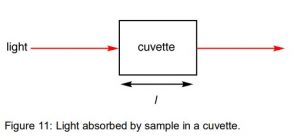
The power of the light going through the example cell is additionally estimated for that frequency – given the image, I. In the event that I am not as much as Io, the example has retained a portion of the light (disregarding the impression of light off the cuvette surface).