Limits and Continuity Explained

Summary:

This comprehensive note provides a detailed explanation of limits and continuity in calculus, covering a range of topics, including tangent and velocity problems, the limit of a function, infinite limits, limit laws, the squeeze theorem, continuity, the intermediate value theorem, and continuity theorems. The note includes examples to aid in understanding and application of these concepts.

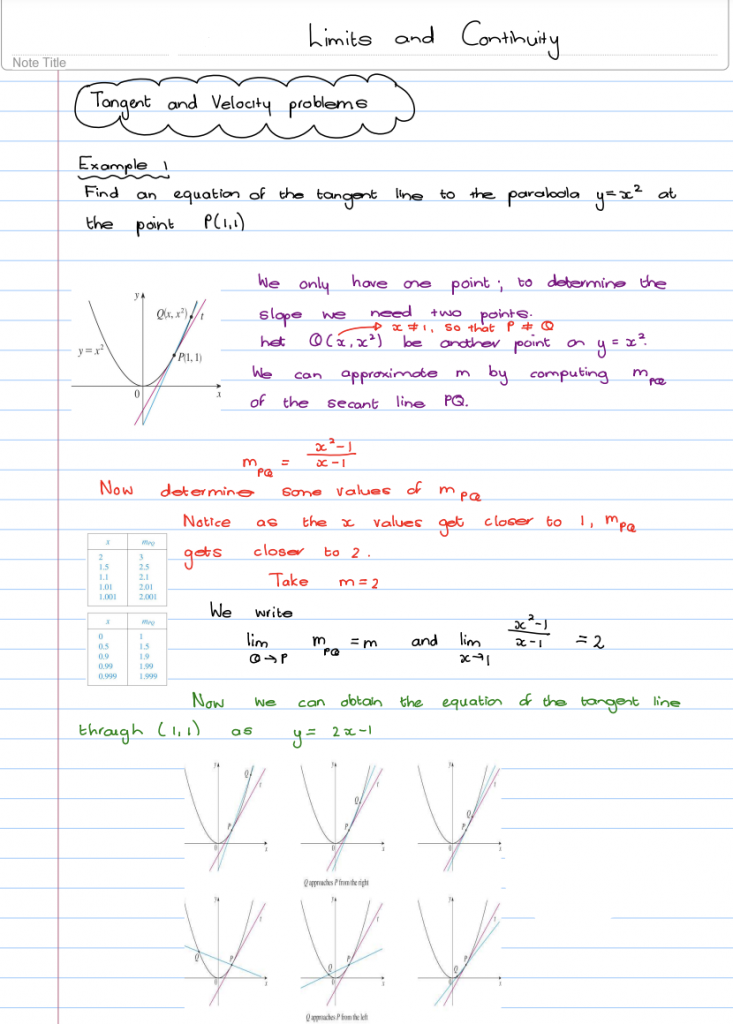
The note begins by introducing tangent and velocity problems, which involve finding the instantaneous rate of change of a function at a specific point. It highlights the significance of limits in solving these problems and lays the foundation for understanding the limitations of a function.

The concept of limits is then explained, emphasizing the idea of approaching a specific value as the independent variable gets arbitrarily close to a particular point. The note provides examples and techniques for calculating limits using limit laws, which allow for evaluating the limits of algebraic functions through algebraic manipulations.

Infinite limits are explored, where the value of the function approaches positive or negative infinity as the independent variable approaches a particular point. The note explains the behaviour of functions near vertical asymptotes and provides strategies for determining infinite limits.

The squeeze theorem, also known as the sandwich theorem, is introduced as a powerful tool for evaluating limits. It states that if two functions “sandwich” a third function and have the same limit as the independent variable approaches a particular point, the third function also has that limit.

Continuity is discussed in detail, emphasizing the connection between limits and continuity. The note explains the conditions for a function to be continuous at a point and explores the concepts of removable and non-removable discontinuities.

Excerpt:

Limits and Continuity Explained

This note offers a comprehensive exploration of limits and continuity in calculus. It covers tangent and velocity problems, the limit of a function, infinite limits, limit laws, the squeeze theorem, continuity, the intermediate value theorem, and continuity theorems. The inclusion of examples enhances understanding and facilitates the application of these concepts in solving mathematical problems.