Intro to Calculus Integration (Grade A+)

Summary:

The PDF titled “Integration Notes” serves as an introductory guide to the concept of integration in calculus. It begins by defining integration as the reverse process of differentiation, used for finding the accumulation or total of a quantity over a given interval. The document distinguishes between Definite and Indefinite Integrals. Indefinite integrals represent a family of functions and are denoted as ∫f(x) dx. Definite integrals, denoted as ∫[a, b] f(x) dx, represent the net area between the function and the x-axis over a specific interval. Various techniques for integration are discussed, including Basic Rules like Linearity and Constant Multiple, Substitution Method (u-substitution), Integration by Parts, Trigonometric Integrals, and Partial Fraction Decomposition.

The notes also delve into integration applications, such as calculating the area under curves, finding the volume of a solid formed by rotating a curve, determining work done by a force, and calculating probabilities. Other applications include finding the arc length and surface area generated by revolving a curve and dealing with improper integrals over unbounded intervals or functions with vertical asymptotes. The document concludes by emphasizing the importance of practice and understanding the context in which integration is applied. It suggests that mastering integration is crucial for various fields in mathematics and science (Pages 1-2).

Excerpt:

Intro to Calculus Integration

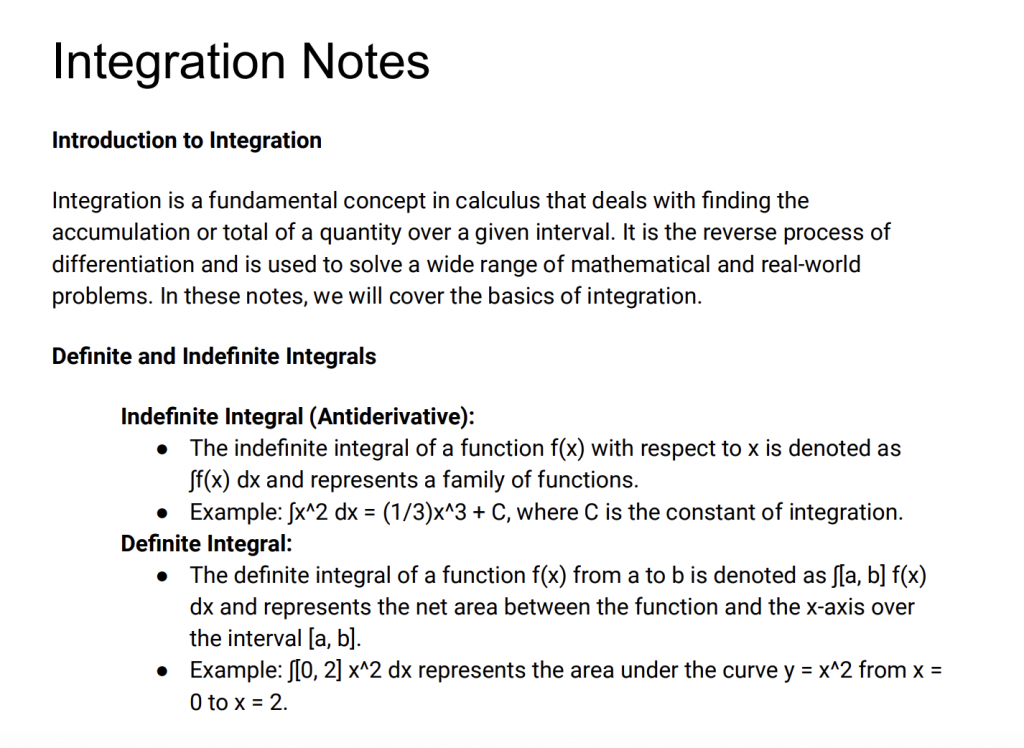
Integration Notes

Introduction to Integration

Integration is a fundamental concept in calculus that deals with finding the accumulation or total of a quantity over a given interval. It is the reverse process of differentiation and is used to solve a wide range of mathematical and real-world problems. In these notes, we will cover the basics of integration.

Definite and Indefinite Integrals
Indefinite Integral (Antiderivative):
● The indefinite integral of a function f(x) with respect to x is denoted as ∫f(x) dx and represents a family of functions.
● Example: ∫x^2 dx = (1/3)x^3 + C, where C is the constant of integration.